The Fermi Function Lesson

by Greg Lush

Search

Search pages

Wiki

Tools

Using The Fermi Function

The Fermi function is a probability distribution function. It can only be used under equilibrium conditions. The Fermi function determines the probability that an energy state (E) is filled with an electron when the material we are working with is under equilibrium conditions. The Fermi level (EF) helps determine carrier distributions. To a first approximation, all the energy states above the Fermi level have a low probability of being filled with electrons and all the energy states below the Fermi level have a high probability of being filled with electrons. For an electronic state with energy the same as EF, the probability of that state being filled is 1/2, or 50%.

Some of the properties of the Fermi function are:

The probability an energy state is occupied: 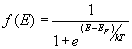image29.gif

The probability an energy state is empty: 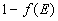image32.gif

The Fermi function at E = EF: f (EF) = 1/2

The Fermi function is simply a mathematical function and has no units: 0 < f (E) < 1

In a band diagram, the position of the Fermi level determines which carrier dominates. If the semiconductor contains more electrons than holes, n-type material, the Fermi level is positioned above mid gap. If holes are more abundant than electrons, p-type material, EF is positioned below mid gap. When the electron and hole concentrations are approximately equal, intrinsic material, EF is positioned at mid gap. The Fermi function, or level, also varies with temperature and carrier concentration.

Created on , Last modified on