Objective of Lesson: To be sure you know how to derive the ideal diode equation, that you know what “ideal” is, and that you are aware of the assumptions needed to derive the equation.
Reading Assignment: Sections 6.1.2 and 6.1.3
Discussion Questions:
1. What are assumptions?
2. What assumptions are needed to derive the ideal diode equation?
3. Why do we have to assume no recombination or generation in the space charge region?
4. What is the Law of the Junction?
Homework: Problem 6.1 from the textbook.
What do you need to know for the exam?:
1. How to do the derivation
Summary
The derivation of the ideal diode equation is not unlike previous work with the minority carrier diffusion equations. This lesson expands on the derivation in the text, but the text’s derivation should be sufficiently clear for the student.
Ideal Diode Equation
We have used the minority carrier diffusion equations to solve problems in semiconductors before so this will be nothing new with a couple exceptions.
The situation is described here…
1. The diode is in steady-state (the voltage has been on for a long time).
2. Diode is a step junction.
3. There is no generation (GL=0).
4. All of the assumptions needed for being able to use the minority carrier diffusion equations are valid
a. Low-level injection holds everywhere.
b. Semiconductor is non-degenerately doped.
c. The problem is one-dimensional.
d. Electric field is zero in the neutral regions.
e. Doping is uniform.
f. We are only working with minority carriers.
5. No recombination or generation in the space charge region.
The text shows the derivation in sufficient detail. Let’s talk about some of the new things.
One of the key new aspects of the derivation is the Law of the Junction boundary condition. The reason it is referred to as a “Law” is that it is true no matter what the circumstances. If for instance we are interested in deriving a current-voltage equation for a solar cell, there will be light inside the device, and that light will generate electron-hole pairs near and inside the junction—but the Law of the Junction as a boundary condition will still hold.
The second new thing is that there is no recombination or generation in the space charge region. The result of this assumption is that the electron current on one side of the space charge region is the same on the other side—and the hole current on one side of the space charge region is the same on the other side. This assumption, it will turn out later, is not valid, but it is valid for this mechanism. Recombination and generation in the space charge region are two additional mechanisms that result in charge crossing the junction so they create additional current as measured externally, but these current mechanisms are in parallel with the ideal diode current; they do not conflict or compete with the ideal diode current.
This comes up in Section 6.1.2 where equations 6.7 – 6.9 are presented.
The rest of the derivation should be familiar to you, and any student should be able to perform this derivation. The result is,
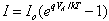
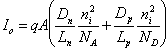 .
.
Note that there are four terms for current—just as there were four mechanisms for charge to cross the junction. Looking at Io (which is called the saturation current), one can see the first term is for electrons (note subscripts and that ni2/NA is the calculation for minority electrons) and the second is for holes. Looking at the term, we can see the diffusion term, and the drift term, -1. Remember we figured out before that the drift current must be a constant—and lo and behold it is so. Note that the diffusion term grows exponentially for positive voltages and goes to zero for even very small negative biases.
These are all the properties we expected based on our qualitative discussion of the diode and its current mechanisms.
The next lesson will explore how changing controllable parameters affects the current observed.